Using Volumetric Flow to Scaleup Chromatographic Processes

When cGMP material for clinical trials and the market is produced, the lab-scale purification process is scaled up based on methods acceptable to authorities in the various countries, including FDA. The transfer of chromatographic processes from lab scale to pilot and production scale is an important and challenging task in the pharmaceutical industry, and has been dealt with in several more or less obvious ways.

Steffen Kidal

Traditional scaleup only expands the column diameter, keeping column length and linear velocity constant, because it is considered a safe procedure.¹ This way of scaling up corresponds to running a number of identical lab columns in parallel, so no wonder it works. However, this principle puts limitations on the development phase, where final production scale demands constraints on column length. Due to wall effects a given minimum column diameter must be maintained.² The wall effects will be reduced as the number of adsorbent particles across the diameter increase, and 200 particles is recommended as a minimum in general practice.

To no one's surprise, lab-scale experiments are performed in unnecessarily long columns, demanding relatively large amounts of product. In certain cases this may impose problems such as lack of sufficient product and safety issues in case of hazardous samples. We let equipment control development when we really should have development control the equipment.

Steffen Kidal, Camilla Kornbeck, and Thomas Hansen in the pilot plant

At manufacturing scale, integration of the consecutive steps may be prevented by rigid constraints on column lengths. The primary way to adjust column capacity is by changing diameter, which means a wide column; or one can multiply the number of columns. In either case flexibility is limited to choosing an integral number of columns. Furthermore, commercial columns are only available with discrete diameters. One more detail; scaling up column diameter without other adjustments may also result in bed-stability problems due to loss of wall support when exceeding a certain diameter-to-length ratio.³

Flexibility in scaleup can be achieved by employing the principle of scaling on a volume basis.⁴ This principle is based on the theory that the residence time for the target protein controls the separation and thus must be kept constant during scaleup. As a consequence the bed height may be increased as long as the flowrate in units of column-volumes/time and load in g/column-volume are kept constant. Increasing column length by this procedure will result in equal or better separation. Scaling down on a volume basis by reducing bed height will, on the other hand, maintain or reduce the plate numbers and may thus compromise the separation (Figure 1).

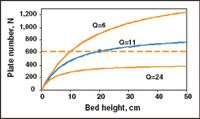
Figure 1. The dotted line represents the minimum plate number required for the separation. In this example 610 plates are needed. At a flow rate(Q) of 11 CV/h the minimum bed height is 20 cm. Reduce the flowrate to Q = 6 CV/h and the minimum bed height is approximately 10 cm. At Q = 24 CV/h separation is not possible at any bed height. At constant Q (fixed residence time) the plate number is always maintained or increased with increased bed height. Thus, for a fixed flow rate the separation is the same or better when the bed height is increased.

Residence time as the controlling factor for dynamic (binding) capacity for protein A affinity chromatography has been reported, and an increased capacity was found.^5,6 This can be explained by the increased number of plates gained by increasing residence time (improved mass transfer and thus a steeper break through curve).

SCALEUP ON A VOLUME BASIS

For a chromatographic process the retention volume (V_R) is a critical parameter. It is crucial to control the retention volume during scaleup, and for this to be achieved it will be necessary to adjust for the difference in extra column effects (dead volume and delay volume) for the involved systems.

Our approach for a flexible and practical method for scaleup uses the principle of scaling on a volume basis while taking extra column effects into account. This makes scaleup a two-step operation:

• Scale up the column process on a volume basis

• Correct for extra column effects created by instrumentation and auxiliaries.⁷

Scaling Equations

Scaling up is based on Equation (1), the van Deemter equation:

H = A + B/v + Cv.......... (1)

In gas systems the B term can be important due to significant molecular diffusion, but in liquid systems B is for all practical purposes negligible.⁸ Thus simplify Equation (1) to Equation (2).

H = A + Cv.......... (2)

The total number of theoretical plates of a column is given by Equation (3):

N = L/H.......... (3)

The efficiency of the column (an increase with decreasing H) is only a function of linear velocity, and that the separation, which depends on the total number of plates, is conserved when maintaining the L/H ratio. The traditional approach has been to conserve H by keeping linear velocity constant, and as a consequence L must be constant.¹ The two equations indicate that for a given N, one could vary L and H arbitrarily as long as the ratio is maintained.

This is, however, not necessarily the case. Introduce a new parameter Q, flow in CV/h, defined in Equation (4).

Q = v/L.......... (4)

Equation (3)may be rewritten. Plug Equation (2) into Equation (3). Then eliminate v with Equation (4) to arrive at Equation (5).

It is informative when N is expressed as a function of bed height and flowrate (in column volumes/time). Figure 1 is a plot of plate numbers as a function of bed height at different flowrates. We immediately see that increased bed height will always result in an increased plate number and thus better or equal separation. As the bed height increases further, an asymtotic plate number is reached (N approaches 1/CQ).

According to Equation (4) an increase in L must be accompanied by an increase in v in order to maintain constant Q. Therefore, when scaling up on a volume basis, v cannot be constant.

However, Figure 1 also shows another important aspect: Scaling down (reducing column length) will result in worse or unchanged separation because N is reduced. As a consequence it is important during process development to find and operate the shortest possible column suitable for the separation. This has two benefits: reduced demand for test material during development and a safely up-scalable process.

To summarize the rules of obtaining flexibility during scaleup:

• Maintain constant flowrate Q in CV/h (not v in cm/h)

• Minimize bed height for successful separation

• Maximize bed height while keeping pressure drop reasonable.

Since the cycle time is constant (constant CV/h) the productivity [g/L_gel/h] is constant, therefore the capacity [g/h] can be adjusted by changing the bed height.

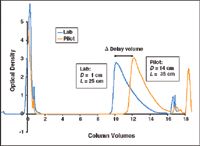
Figure 2. Example of scale up on a volume basis from lab to pilot and in pilot (different bed heights and diameters). Q = 12 CV/h.

EXTRA COLUMN EFFECTS

An example of scaling up on a volume basis directly from lab-scale to pilot-scale (by a factor 274) is shown in Figure 2. For all experiments we use columns 20 to 35 cm long. The 20-cm column length was measured as 610 plates at Q = 11 CV/h. As both experiments showed identical elution and impurity profiles we have obtained the same separation and thus successfully scaled the process. However, the transfer from lab to pilot resulted in a significant larger retention volume. This deviation arises from extra column effects.

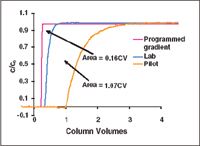
Figure 3. Areas equal the dead volumes of different systems, 0.16 CV for the lab and 1.07 CV for pilot. c/c0 is the scaled concentration

The deviation of 2.3 CV was initially attributed to a difference in the dead volumes. These were calculated by numerical integration as the area between the nominal gradient (programmed gradient) and the lab or the pilot gradient (Figure 3) and found to be 0.16 CV and 1.07 CV respectively. This difference of 0.91 CV, obviously did not account for the deviation.

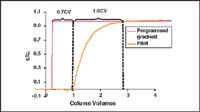
Figure 4. The pilot response curve shows that the delay volume (2.6 CV) is made up by two contributions, one from tubing (0.7 CV) and one from mixer (1.9 CV).

Next, by measuring the gradient response curves with columns off line in lab and pilot the total delay before matching the nominal gradient could be determined. Figure 4 shows the gradient response curve for pilot with the resulting delay volume of 2.6 CV. The corresponding result for lab scale was 0.3 CV. The difference in delay volumes from pilot and lab (Δ delay volume) could now be determined as 2.3 CV as shown in Figure 5, which accounts for the deviation.

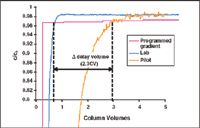
Figure 5. Example of measurement of extra column effects (Δ delay volume = 2.3CV).

The general relationship between delay volume and gradient slope (G) is shown in Figure 6. In the extreme situation of zero slope (isocratic elution) the delay volume will in theory be infinite as the response curves will never reach the nominal gradient, but in a practical approach the delay may be chosen as the difference at 95% match.

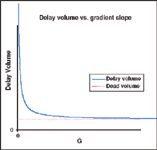
Figure 6. Delay volume vs. gradient slope (G)

For very steep gradients, on the other hand, the delay volume will approach the dead volume. Therefore, in most cases it will be necessary to apply corrections for the actual delay volume differences in order to obtain matching retention volumes.

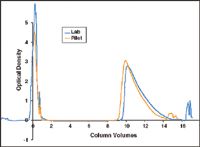
Figure 7. Profiles from Fig. 2 corrected for the Δ delay volume shown in Fig. 5. Note that the profiles do not match completely due to the better performance (slightly higher and narrower main peak) in pilot scale as predicted by the increased number of plates. The differences in the tailing edge are caused by different regeneration protocols (for column clean in place) used in lab and pilot.

In Figure 7 the elution profiles from Figure 2 are corrected for the 2.3 CV difference in delay volume. The residual difference in retention volume may be explained from the different gradient responses in lab and pilot runs (Figure 5).

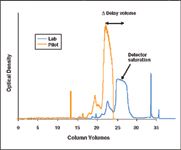
Figure 8. Scaleup example with a different set of materials: Lab: 1 x 25 cm = 20 mL, 6 CV/h (150 cm/h). Pilot: 20 x 35 cm = 11,003 mL, 6 CV/h (210 cm/h). The profiles would be the same height but the detector in the lab saturated.

An entirely different scaleup example is shown in Figure 8. Delay volume causes the difference in retention volumes. The compressed shape of the lab profile is due to UV-detector saturation. In Figure 9 the profiles are compared, corrected for Δ delay volume thereby confirming this method.

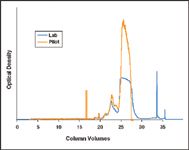
Figure 9. Profiles from Fig. 8 corrected for D delay volumes. Note that the profiles do not match completely due to the better performance (slightly narrower main peak and better separated peaks) in pilot scale as predicted by the increased number of plates.

APPLICABILITY

In order to predict and correct for differences in retention volumes when scaling up a particular process from lab to pilot and production scale a table of Δ delay volumes for the process in all three systems was constructed. The results are shown in Table 1, which also contains the dead volumes for comparison.

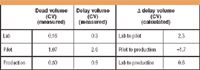
Table 1. Sample table of delay volumes (for the relevant gradient). Retention differences can be predicted and corrected for during scaling up (or down) by applying the D delay volume. The table could be used to directly transfer a process between lab and production.

We have shown that it is possible to scale up the chromatographic process at constant productivity while changing both the bed height and the diameter. In both experimental cases identical impurity profiles were found at scale up. The differences in retention volumes observed during process transfer from lab to pilot could be accounted for by extra column effects. This requires using the delay volume from the relevant gradient (and not just the dead volume) for correction.

ACKNOWLEDGEMENTS

We would like to thank Ernst Hansen (Novozymes) for scientific discussions and review and Lars Sejergaard (Novo Nordisk) for collaboration with ideas and experiments.

Steffen Kidal is a research scientist, 201 BioProcess Purification at Novo Nordisk A/S (Brudelysvej 20, DK-2880 Bagsvœrd, Denmark, 45.4443.2545, sffk@novonordisk.com).

Ole Elvang Jensen is principal scientist, 427 Purification Plants at Novo Nordisk A/S (Hagedornsvej 1, DK-2820 Gentofte, Denmark, 45.4443.9279, oej@novonordisk.com)

REFERENCES

1. Amersham Pharmacia Biotech (now GE Healthcare). Ion Exchange Chromatography – Principles and Methods. Uppsala, Sweden; 2001 p.121

2. Wancat PC. Rate Controlled Separations. London; Chapman & Hall; 1994. p. 330

3. Stickel JJ and Fotopoulos A. Pressure-flow relationships for packed beds of compressible chromatography media at laboratory and production scale. Biotechnol. Prog. 2001; 17(4):744-751.

4. Hansen. E. Scale up on a volume basis – Theory and applications [thesis]. Novo Nordisk A/S, Copenhagen, Denmark; May 2002. (Private communication).

5 Fahrner RI, Iyer HV, Blank GS. The optimal flow rate and column length for maximum production rate of protein A affinity chromatography. Bioprocess Engineering 1999; 21:287-292.

6 McCue JT, Kemp G, Low D, Quinones-Garcia I. Evaluation of protein-A chromatography media. J. Chroma. A. 2003; 989:139-153.

7. Gagnon P. Avoiding instrument-associated aberrations in purification scale-up and scale-down. BioPharm 1997 March; 10(3):42-45.

8. Wancat PC. op. cit. p.328.

Definitions

A = Axial dispersion parameter (band broadening which contributes to plate height, constant for a particular case), cm

B = Molecular diffusion, cm² /h

C = Mass transfer parameter (band broadening which contribute to plate height, constant for a particular case), h^-1

c = Concentration at column outlet, M

c₀ = Concentration at column inlet = load concentration, M

CV = Number of column volumes, dimensionless

G = Gradient slope, M/CV = M

Gradient response = The resulting UV-gradient curve from a programmed (nominal) gradient.

H= Plate height (a smaller number results in a sharper chromatographic peak and thus a more efficient column), cm

h = Time unit, hour

L= Bed height, cm

M = Molar concentration, mol/L

N= Plate number (the total number of plates in a column; higher number means longer column, results in better separation)

Q= Flowrate in column-volumes/time, CV/h

v= Linear velocity, cm/h

V_R = Retention volume, (the number of column volumes at which the product elutes), CV

Δ delay volume = The difference in delay volumes between different systems (lab, pilot and production)