Using Direct Transition Analysis in Chromatography

science photo/Shutterstock.com

Abstract

The authors present a robust and easy-to-implement chromatography column performance assessment method, called direct transition analysis (DTA). Because conventional transition analysis based on moment analysis is often challenging to validate and implement in good manufacturing practice (GMP) operations, a “paper-and-pencil” method for production-scale column performance qualification and monitoring was developed. DTA results were found to be in good agreement with moment analysis. The authors demonstrate the simplicity of DTA calculation and its successfull implementation in a GMP environment through three case studies covering column re-qualification, resin lifetime process validation, and column routine performance monitoring.

Introduction

Consistent and controlled chromatography column performance is crucial to ensuring product quality for commercial production of recombinant proteins. One method applied to production-scale chromatography columns is called pulsed-input (salt-plug) testing (1-4).

This method is typically used to evaluate a freshly packed column where a chemical tracer (typically salt or base solution) is injected, and an elution peak with a Gaussian (normal) distribution is generated.

The height equivalent of a theoretical plate (HETP) and asymmetry factor (Af) can be extracted from the Gaussian curve. However, this method is less practical for ongoing monitoring of production-scale chromatography columns in routine manufacturing operations because it is severely limited in sensitivity, needs installation of auxiliary equipment, and is too cumbersome to carry out on a routine basis and obtain results in a robust way.

Another method called moment analysis (5), first applied to chromatography by Martin and Synge (6), is an alternative column assessment tool to the pulsed-input testing method. The analysis is performed on a chromatography transition, which is a response at the column outlet to a step-change at the column inlet.

The derivative of the response curve is a peak-like curve, but not necessarily Gaussian distribution. The HETP and Af can then be calculated based on the derivative curve as described in the materials and methods section in this paper.

The transition is typically a result of buffer change as part of the normal operation recipe, thus no dedicated tracer study is needed, and moment analysis can be performed on the in-process data during or after each batch, without affecting the normal column operation. Thus, it is more practically applied to production-scale chromatography columns in operation (7, 8).

Larson et al. (7) performed moment analysis to assess more than 300 production-scale chromatography transitions. They found that the non-Gaussian HETP method is much more sensitive in detecting bed integrity breaches compared to the Gaussian HETP method (moment analysis with the assumption of Gaussian distribution).

Because moment analysis is performed on the derivative of the sensor data (typically conductivity), which amplifies the noise in the original data, it often requires extensive signal processing or noise filtering, e.g., change band or moving average filter (7, 8), highly depending on the signal-to-noise ratio of sensor data (as illustrated in Figure 1). There are no established standards or best practices on how data should be pre-processed. Different columns require different signal processing approaches, which would thus lead to different results in HETP and Af.

Figure 1. (a) Comparison between Af from moment analysis (7) and DirectAf from direct transition analysis (DTA) calculated over normal campaign runs of an affinity chromatography column where same-column operations were executed consecutively with little or no pause. (b) The derivative of conductivity data versus column volume (CV) with and without noise filtering from one of the campaign runs.

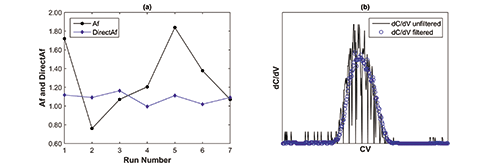
Figure 1. (a) Comparison between Af from moment analysis (7) and DirectAf from direct transition analysis (DTA) calculated over normal campaign runs of an affinity chromatography column where same-column operations were executed consecutively with little or no pause. (b) The derivative of conductivity data versus column volume (CV) with and without noise filtering from one of the campaign runs [All figures are courtesy of the authors].

To address this challenge, a “paper-and-pencil” method, which the authors named direct transition analysis (DTA), was developed to overcome the shortcoming of moment analysis. Two column performance indicators, transition width (TransWidth) and direct asymmetry factor (DirectAf), corresponding to HETP and Af, respectively, are directly extracted from the transition curve without complex signal processing.

The main drive behind this approach is to pave the way for validation, which is a regulatory requirement for commercial manufacturing. The calculation procedure of these two metrics and their applications to production-scale chromatography columns are presented in this paper.

Materials and methods

Chromatography data

In-process data from production-scale chromatography column operations for recombinant protein productions were used for conducting moment analysis and DTA. The chromatography columns were either bind-elute or flow-through covering various affinity chromatography, hydrophobic interaction chromatography (HIC), and ion exchange chromatography (IEX) columns. Conductivity was chosen as a measure of transition. When switching buffers during normal column operation, a step-change in conductivity was introduced at column inlet, and a transition occurred as a response to the step-change at column outlet. Thus, the post-column conductivity data were collected and used for both analyses.

Typically, the transition was chosen at non-product-related phases with large conductivity differences, preferably before loading to the column. Flow-rate data were also used for integration over time to obtain column volume (CV) during the transition. A transition curve was obtained by plotting the post-column conductivity data versus CV, instead of time, to eliminate the impact of operation delays on analysis results. Although DTA is functional for lower frequency data (up to 20-second interval), higher frequency (≤ five-second time interval) post-column conductivity and flow-rate data were desired to ensure reliable analyses.

Moment analysis

Moment analysis was conducted based on the theories from the transition study on production-scale chromatography data by Larson et al. (7). Two metrics, non-Gaussian HETP and Af, were calculated based on the smoothed derivative of post-column conductivity data versus CV over clearly defined transition phases. The detailed calculation procedure is shown below:

• The volume (V) passing through the column was converted from liter to CV.

• Conductivity (C) was normalized to 0 (min.) ~1 (max.).

• Normalized conductivity was plotted against CV.

• The CV was obtained when conductivity crosses the 0.5 threshold, CV_mid.

• Conductivity data were renormalized between CV_mid ± 0.35 CV as the transition window.

• Normalized conductivity was interpolated to handle large gaps and super clusters.

• The derivative of the conductivity data was taken and the noise was filtered using Baxter-King band pass filter (9).

• The non-Gaussian HETP based on statistical moments, M_i, and the column bed height, H, was calculated using Equation 1 (10):

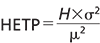
[Eq.1]

where:

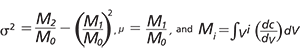
Af was calculated using Equation 2:

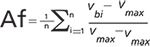
[Eq. 2]

where V_max is the value of V corresponding to (dC/dV)_max, and V_bi and V_ai are the value of V at x% of (dC/dV)_max, V_bi > V_ai. While single threshold of 10% (n=1 and x=10) was commonly used for moment analysis (e.g., Larson et al.’s work [7]), multiple thresholds (n=6 and x=10, 20, 30, 40, 50, and 70) were implemented on the noisy manufacturing process data shown in this paper in order to mitigate the risk of single-point failure.

Direct transition analysis (DTA)

A challenge in the moment analysis is that it usually requires extensive signal processing or noise filtering, because the analysis is performed on the derivative of the conductivity data, which aggravates the noise in the original data. For legacy equipment, it is not uncommon that the signal-to-noise ratio of derivative signal is so poor that moment analysis fails to generate reliable results. Because of this, a “paper-and-pencil” approach, DTA, was developed.

Corresponding to HETP and Af in the moment analysis, two metrics from DTA, TransWidth and DirectAf, were extracted directly from the transition curve of post-column conductivity versus CV without extensive signal processing. TransWidth is a direct measure of band broadening of the column, while DirectAf is a direct measure of asymmetry. As a pre-requisite, moment analysis requires a time stamp at the beginning of buffer change. In real-world application, it can be difficult, depending on data historian setup, for the chromatography skid. DTA, however, does not need the precise timing of buffer change. A detailed calculation procedure (illustrated in Figure 2) is shown below:

• The volume passing through the column was converted from liter to CV.

• Conductivity was normalized to 0 (min.) ~1 (max.).

• Normalized conductivity was plotted against CV.

• The CV was obtained when conductivity crosses the 0.5 threshold, CV_mid.

• Conductivity data was renormalized between CV_mid ±0.35 CV as the transition window.

• The CVs when conductivity crosses multiple thresholds were obtained, respectively.

• TransWidth was calculated as the difference between CVs at thresholds of 95% and 5%.

• DirectAf was calculated as the average of all bi/ai that were obtained at multiple thresholds as shown in Figure 2.

Figure 2. Illustration of TransWidth and DirectAf calculation. Here is an example of up transition in which new buffer has higher conductivity.

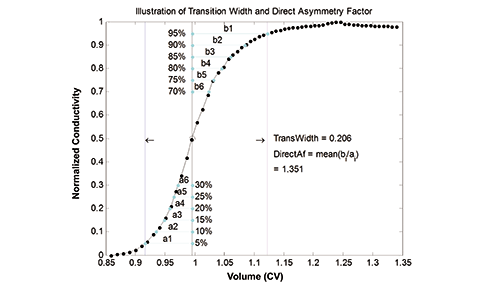
Figure 2. Illustration of TransWidth and DirectAf calculation. Here is an example of up transition in which new buffer has higher conductivity.

Results and discussion

Comparisons between moment analysis and DTA

Comparisons of results from moment analysis and DTA for production-scale chromatography columns are shown in the following examples. The first example demonstrates the challenge of implementing moment analysis on manufacturing process data due to the noise in sensor data requiring extensive noise filtering.

The advantage of using DTA is that it is much less impacted by noise and requires no special filtering, therefore, it is easier to implement. To fully demonstrate signal noise effects, moment analysis in this example followed Larson et al.’s work (7) using moving average filter to smooth the derivative of conductivity data and only 10% threshold (n=1 in Equation 2) to calculate Af.

Figure 1(a) shows the comparison between Af from moment analysis (7) and DirectAf from DTA calculated over several affinity chromatography column runs during normal operations within a campaign where same-column operations were executed consecutively with little or no pause. It is seen that the result from DTA showed more consistency than the one from moment analysis (7) under normal operation conditions.

Figure 1(b) shows the derivative of conductivity data versus CV with and without noise filtering from one of the campaign runs illustrating how noise in original conductivity data can be amplified when taking derivative and complex filtering is necessary for moment analysis.

The second example shows the comparison of results from moment analysis and DTA described in the materials and methods section of this paper and further exploration of the relationship between the two sets of metrics from both analyses. All metrics were calculated based on one-year historical data from a HIC column.

Results are compared in Figure 3. HETP and TransWidth have -similar trends (though they have different units in terms of processing length or volume), and Af and DirectAf have similar trends as well. There are distinct offsets between the moment analysis and direct analysis, but the main objective of these analyses is to detect the shift in column performance. In real-world application, acceptance criteria could be established using proper statistical rules, instead of focusing on the absolute values.

Figure 3. Comparisons and correlations between moment analysis and direct transition analysis (DTA) metrics over one-year historical lots for a hydrophobic interaction chromatography (HIC) column. HETP is the height equivalent of a theoretical plate. CV is column volume.

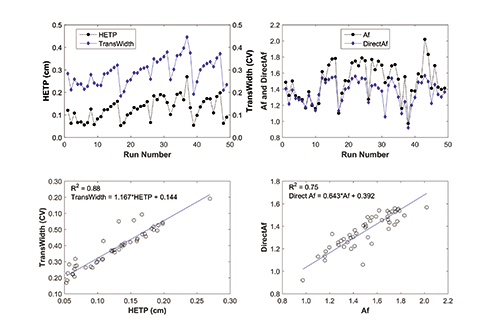
Figure 3. Comparisons and correlations between moment analysis and direct transition analysis (DTA) metrics over one-year historical lots for a hydrophobic interaction chromatography (HIC) column. HETP is the height equivalent of a theoretical plate. CV is column volume.

To further check the relationship between the two sets of metrics, correlations were performed as shown in Figure 3. Results indicate there are strong linear correlations between HETP and TransWidth (coefficient of determination R²=0.88), and between Af and DirectAf (R²=0.75). Therefore, TransWidth and DirectAf could be good substitutes for HETP and Af.

Case study 1: Chromatography column re-qualification

DTA was used to re-qualify production-scale chromatography columns after a facility upgrade required relocation of multiple previously qualified columns. Blank runs (without loading products) were performed after the columns were moved back to the suite. The conductivity and flow-rate data from those runs were collected and analyzed using DTA to assess the integrity of the column. For each column, the TransWidth and DirectAf were calculated for the blank runs as well as over the life of the current column pack on which acceptance criteria were established. The acceptance criteria, or the single-value prediction interval, were calculated as [LPL, UPL] using Equation 3:

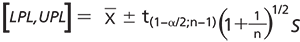
[Eq. 3]

where LPL and UPL are the lower and upper prediction limits, respectively, xˉ is the sample mean, s is the sample standard deviation, n is the sample size, and t_(1α/2;n-1) is the two-sided t-distribution statistic with n-1 degrees of freedom (α=0.05).

During the relocation, one IEX chromatography column experienced physical impact. TransWidth and DirectAf from its blank run were 0.199 and 1.180, -respectively. The single-value prediction interval for TransWidth was [0.202, 0.238] and the single-value prediction interval for DirectAf was [0.913, 1.312]. As seen in Figure 4, the TransWidth value was outside the interval, and thus failed to meet the acceptance criteria, leading to a column re-packing. Other columns for which the metrics from the blank runs met the criteria were considered re-qualified and were released for production.

Figure 4. TransWidth and DirectAf trends for ion exchange chromatography column runs including the blank run. The dash lines represent the acceptance criteria based on Equation 3. CV is column volume.

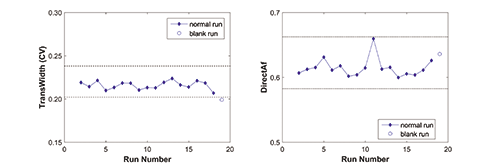
Figure 4. TransWidth and DirectAf trends for ion exchange chromatography column runs including the blank run. The dash lines represent the acceptance criteria based on Equation 3. CV is column volume.

Case study 2: Chromatography resin lifetime process validation

DTA was used to support chromatography resin lifetime process validation (PV). TransWidth and DirectAf were chosen as lifetime indicators of the resin bed integrity. Both metrics were calculated for each column cycle and monitored throughout its intended use period. Figure 5 shows TransWidth and DirectAf trends through the resin lifetime PV for an affinity chromatography column. It can be seen that the TransWidth and DirectAf remain consistent among cycles.

Figure 5. TransWidth and DirectAf trends during resin life time process validation for an Affinity chromatography column. The solid line represents the mean value and the dash lines represent the +/-3 sigma range. CV is column volume.

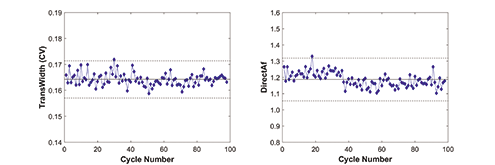
Figure 5. TransWidth and DirectAf trends during resin life time process validation for an Affinity chromatography column. The solid line represents the mean value and the dash lines represent the +/-3 sigma range. CV is column volume.

Case study 3: Chromatography column routine performance monitoring

DTA is used together with other critical process parameters for monitoring chromatography column performance on a routine basis. For example, atypical UV peaks were observed from the most recent two runs of a HIC column. DTA metrics were used to assess whether the column bed integrity degraded in addition to other potential root causes. As shown in Figure 6, a shift in TransWidth and DirectAf trends was observed where both metrics for the most recent two runs failed to meet the acceptance criteria based on Equation 3. Thus, a decision was made to re-pack the column. Later, it was confirmed that preferential flow characteristics was formed inside the packed resin bed and the start of channel formation was observed during column unpacking.

Figure 6. TransWidth and DirectAf trends for a hydrophobic interaction chromatography column. The dash lines represent the acceptance criteria based on Equation 3. CV is column volume.

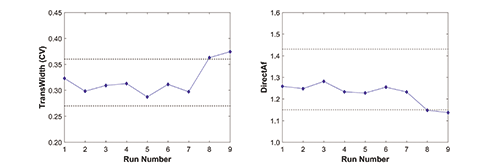
Figure 6. TransWidth and DirectAf trends for a hydrophobic interaction chromatography column. The dash lines represent the acceptance criteria based on Equation 3. CV is column volume.

Conclusion

DTA was demonstrated as a reliable and easy-to-implement approach to qualify and monitor the performance of production-scale chromatography columns. The straightforward calculation algorithm without complex signal processing made DTA easy to implement and the results were less affected by the quality of sensor data. Results from DTA compared against the corresponding ones from the conventional moment analysis showed that TransWidth and HETP, and DirectAf and Af, correlated well, respectively. DTA could be a good substitute for moment analysis, or the two could be used simultaneously to enhance the monitoring and control of chromatography operations.

Acknowledgements

The authors would like to thank Global/Site MSAT staff who contributed to DTA improvements and applications in chromatography productions.

References

1. M. Hofmann, J. Chromatogr. A 796 (1) 75-80 (1998).
2. S. Gondkar et. al, Biotechnol. Prog. 17 (3) 522-529 (2001).
3. J. Moscariello et. al, J. Chromatogr. A 908 (1-2) 131-141 (2001).
4. A. Williams et. al, J. Chromatogr. A 944 (1-2) 69-75 (2002).
5. R.G. Harrison et. al, Bioseparations Science and Engineering, pp.259-264 (Oxford University Press, New York, 2nd ed., 2015).
6. J.P. Martin and R.L.M. Synge, Biochem. J. 35 (12) 1358-1368 (1941).
7. T. Larson et. al, Biotechnol. Prog. 19 (2) 485-492 (2003).
8. C. Bork et. al, Biotechnol. Prog. 30 (2) 383-390 (2014).
9. M. Baxter and R.G. King, NBER Working Paper Series No. 5022, (1995).
10. B. McCoy and M. Goto, Chem. Eng. Sci. 49 (14) 2351-2357 (1994).

Peer-Reviewed

Article submitted: August 16, 2017.

Article accepted: September 26, 2017.

Author Bios

Yuanyuan Cui^*, is process engineer III, Yuanyuan.Cui2@sanofi.com; Zheng Huang is director; and Jack Prior is head, all at Global Manufacturing Science and Technology, Sanofi.
^*To whom correspondence should be addressed.

Article Details

BioPharm International
Volume 31, Number 1
January 2018
Pages 34–40

Citation

When referring to this article, please cite as Y. Cui, Z. Huang, and J. Prior, “Using Direct Transition Analysis in Chromatography,” BioPharm International 31 (1) 2018.